
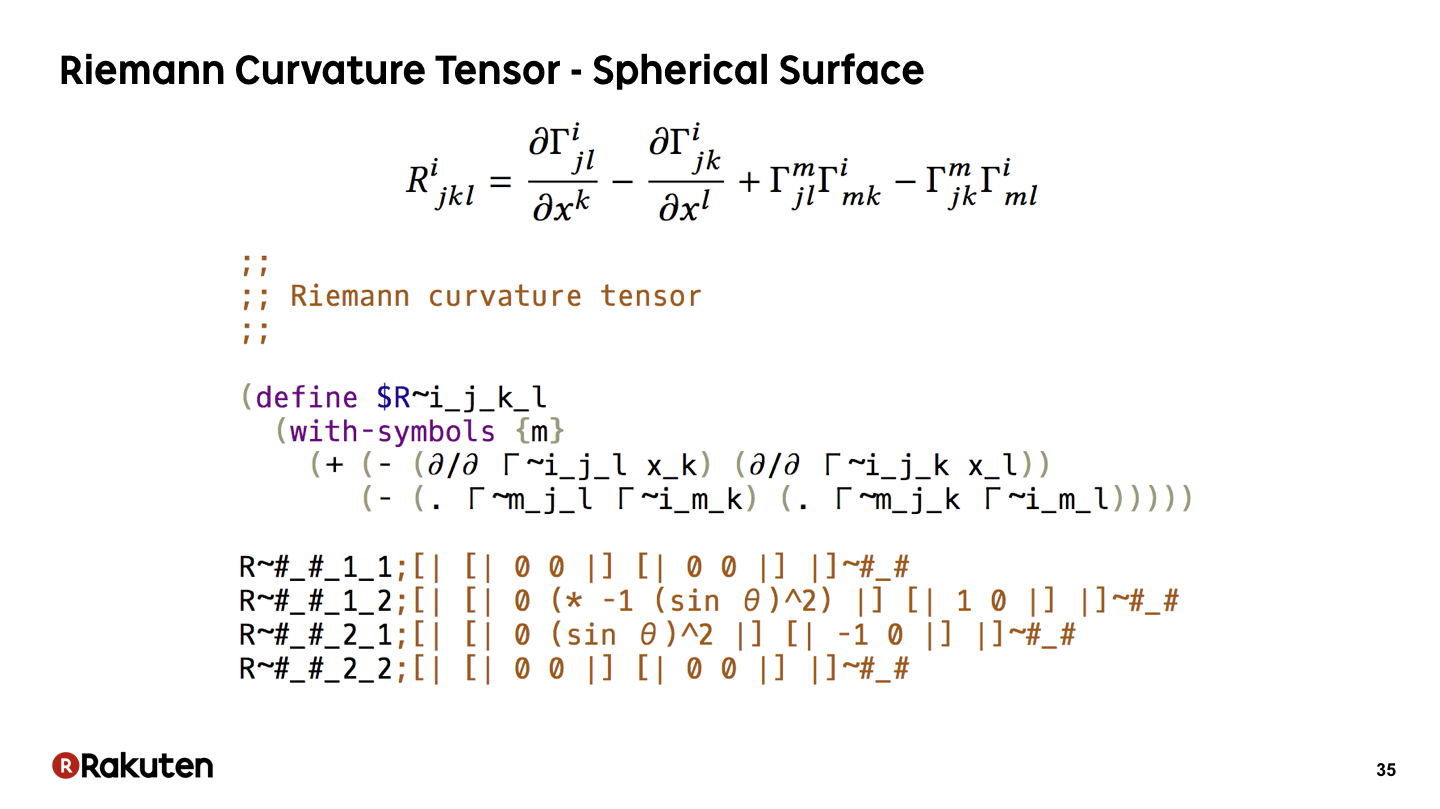
This returns the Christoffel symbol of second kind that represents the Levi-Civita connection for the given metric. $$g=\begin$ (which is annoying, since the article uses my preferred notation which I have made a conscious effort to move away from due to none of my professors using it), and was wondering whether or not I made a fundamental mistake, or if the different labeling systems could account for the discrepancy. metrictoChristoffel2nd (expr) source Return the nested list of Christoffel symbols for the given metric. First fundamental form The metric or rst fundamental form on the surface Sis dened as gij: ei ¢ej. Note that while nis a unit vector, the e are generally not of unit length. So for Einstein’s theory, the metric is enough1. The vectors e(r) belong to TrS, the tangent space of Sat r, this is why we use a dierent notation for them than the ordinary' vectors from R3.

We can easily then see that the metric and the inverse metric are In such space-times, the metric de nes a connection (for us, they will anyway), the connection de nes the Rieman-nian curvature, and the Riemannian curvature is, by Einstein’s equations, related to sources.


 0 kommentar(er)
0 kommentar(er)
